Sherwin and Chapple (1968) based on measurements on naturally developed "single" layer folds, most of these being quartz veins in phyllitic matrix (Sprague Upper Reservoir group), of slaty matrix (Martinsburg slate) or sandy matrix (Vermont Group), found the Wd/t ratios were actually lower than the Biot-Ramberg theory had predicted. The total amplification at the dominant wavelength was also found to be considerably less than that predicted by the Biot-Ramberg theory. In the light of their studies they modified the existing equation

to

where S is the bulk strain ratio involved and which Sherwin and Chapple were able to compute accurately. The equation above is the same as given by Sherwin and Chapple (1968) except that uses the notations similar to that of Biot for immediate ready comparison.

Biot had predicted on the basis of his theoretical analysis, the thin plate theory, that no significant buckling would occur if the viscosity contrast was less than 100:1. Sherwin and Chapple however showed that the dominant wave number ld which is the ratio of 2pt to Wd is an important factor and determines the amount and rate of amplification. Thus folds can form even if the viscosity contrast was just around 4.0. In other words, folds of the same amplitude can be produced under different viscosity contrasts but this will be controlled by the amount of shortening of the material under an assumed plane strain condition.

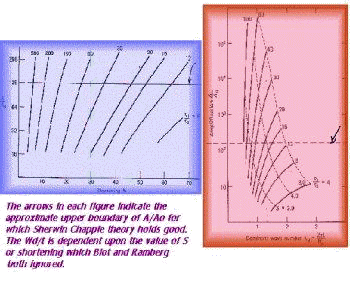
The series of curves for a graph of amplification A/Ao plotted against the dominant wave number 2pt/Wd are shown in one of the figures on this page reproduced after Sherwin and Chapple (1968). Of course it implied that for lower viscosity contrasts, the layer shortening would be predominant, amplification small and this is reflected in the figure reproduced after Sherwin and Chapple.

The original notations by Sherwin and Chapple in the equations quoted above are replaced by those that would draw a logical difference between Biot-Ramberg and this thesis.